| Press Release
Abstract
Monetary policy is often expected to adopt a pro-growth stance in a phase of prolonged slowdown in growth and sluggish investment activities. Sacrificing inflation, i.e. lowering nominal policy rate even when inflation persists at a high level, is a convenient means to lower real interest rates, which in turn could be seen as a pro-growth stance of monetary policy. This paper, using both firm-level and macroeconomic data, and alternative methodologies - such as panel regression, VAR, Quantile regression and simple OLS – finds that for 100 bps increase in real interest rate, investment rate may decline by about 50 bps and GDP growth may moderate by about 20 bps. The empirically estimated sensitivity of investment and growth to changes in real interest rate suggests that if the RBI can lower real lending rates, it can also stimulate growth. Review of literature highlights that a central bank can lower real interest rates either through financial repression or by not responding aggressively to inflation while raising the nominal policy rates in response to inflation. Empirical estimates for India indicate that RBI’s monetary policy response to inflation has not been aggressive, and as a result the Fisher effect –i.e. one for one response of interest rate to inflation that could leave the real rate constant – does not hold. Thus, even when a high nominal interest rate may often signal that monetary policy stance is tight, because of higher inflation and absence of Fisher effect, lower real interest rate may actually be growth supportive. In India, real lending rates in recent years have been generally lower than the levels seen during the high growth phase before the global crisis. But lower real rates in the post-crisis period have coincided with sluggish investment and GDP growth. This is due to the fact that while real rates are lower, marginal productivity of capital, or expected return on new investment has also declined, which has dampened the expected positive impact of lower real rates on investment. In such a scenario, one policy option could be to lower real rates even more, by raising inflation tolerance, i.e. lowering nominal policy interest rate even when high inflation persists or inflation expectations remain high. This paper, however, provides robust empirical justification against any policy of lowering policy interest rates when inflation persists above a threshold level of 6 per cent. The beneficial impact of lower real rates on growth that may be achieved through higher inflation tolerance is more than offset by the harmful effect of high inflation, particularly when it exceeds a threshold level of 6 per cent.
Real Interest Rate impact on Investment and Growth –
What the Empirical Evidence for India Suggests?
I. Introduction
The Reserve Bank had to face and manage a difficult growth-inflation mix in 2012-13, with persistently high inflation requiring resolute anti-inflationary thrust in the conduct of monetary policy on the one hand, and sluggish growth impulses warranting adequate and unambiguous monetary policy stimulus to spur growth on the other. Facing this delicate growth-inflation balance, the Reserve Bank explicitly communicated that: (a) growth sacrifice is a necessary - though unpleasant - means to contain demand side pressures on inflation, (b) high interest rate reflecting anti-inflationary stance of monetary policy is only one of the factors behind the slowdown in growth, and (c) real interest rates, which could be more relevant than nominal interest rate for influencing investment activities and overall economic growth, are lower now compared with the high growth phase before the global crisis, pointing to the role of non-monetary factors in driving and sustaining the slowdown in growth.
In terms of exact communication from the RBI on these aspects, the Mid Quarter Review (MQR) of Monetary Policy dated June 18 2012 highlighted that “... it is relevant to assess as to what extent high interest rates are affecting economic growth. Estimates suggest that real effective bank lending interest rates, though positive, remain comparatively lower than the levels seen during the high growth phase of 2003-08. This suggests that factors other than interest rates are contributing more significantly to the growth slowdown.” Subsequently, in the first quarter review (FQR) dated July 31, 2012, it was stressed that “...While monetary actions over the past two years may have contributed to the growth slowdown – an unavoidable consequence – several other factors have played a significant role. In the current circumstances, lowering policy rates will only aggravate inflationary impulses without necessarily stimulating growth.” This paper was conceptualised against this background, with the aim of empirically examining some of the puzzling policy questions of direct relevance to monetary policy. The relevant questions for monetary policy include whether nominal or real interest rate matters for influencing investment and growth, and can a central bank influence real interest rates? Can a low nominal or real lending rate stimulate investment demand and growth, or whether a supportive non-monetary environment is more important for growth? If sacrifice of the inflation objective is a means to lower real interest rates, can growth be really stimulated through a lower real interest rate, particularly in an environment where inflation is allowed to persist at above the threshold level? No single empirical paper can provide definite answers to all these complex, highly debatable, questions. This paper, however, makes an attempt to seek broad answers to these questions, based on analysis of available macro-economic and firm-level data, using alternative econometric methodologies. In the Third Quarter Review of monetary policy in January 2013, while lowering the repo rate by 25 bps, it was communicated that the expected outcome would include “...support growth by encouraging investment”. The repeated emphasis in monetary policy statements during 2012-13 on the expected relationship between interest rate and investment activity and GDP growth provided the key motivation for this paper. Against this context, the paper is organised into six sections. Section-II sets out some of the theoretical controversies on real interest rate. A short review of empirical literature on sensitivity of investment and growth to changes in real/nominal interest rates is presented in Section-III. Section-IV covers some broad policy inferences that could be derived from a mere look at trends in relevant data, besides a narration of the data used in the study. Empirical estimates derived from use of four alternative methodologies – panel regression, quantile regression, ordinary least squares (OLS) regression and VAR – are analysed in Section-V. Feedback received from representatives of industry and commercial banks during a discussion on the significance of nominal versus real interest rate for investment planning is encapsulated in Section-VI. Concluding observations are presented in Section-VII.
Section-II: Controversies on Real Interest Rate
Real interest rate, being an unobservable variable, and also given that in many different ways the real rate could at best only be approximated using alternative measures of both forward looking and backward looking inflation expectations, theoretical and empirical literature on the subject is replete with controversies. This section aims at presenting only those controversies which are relevant to the broad theme of the paper, i.e. the impact of nominal versus real interest rate on investment and growth.
Can a central bank change real interest rates?
A central bank can change real interest rates in two different ways; first, through financial repression/reforms, and second, through a monetary policy response to inflation in a manner that does not allow the Fisher effect to hold. Financial repression could include measures such as regulated interest rates with explicit or implicit caps on nominal rates – irrespective of the level of inflation, directed lending – preventing cost of funds to reflect underlying risks, captive financing of the government borrowings – either through public ownership of banks or excessive use of moral suasion or bank regulation, high reserve requirements and securities transactions tax, and capital controls that prevent outflows of domestic savings and thereby help maintain low domestic interest rates. According to Reinhart (2012), for about 35 years after the end of World War-II, real interest rates in advanced economies remained highly depressed, reflecting financial repression. Then came the wave of financial liberalisation, and real rates started rising, during 1981 to 2007. Since the global crisis, however, these economies are back to another phase of financial repression, with large monetised financing of fiscal deficits, new forms of repression such as macro-prudential measures, systemic risk regulation, and growing justification in intellectual debates for use of capital controls as a policy tool. In this third phase between 2008 and 2011, negative (or non-positive) real rates were observed in close to half of the observations, and real rates were less than 1 per cent in about 82 per cent of the observations (Table 1). Two important points to notice from this table are: (a) the third phase since the global crisis appears to be the most repressive, in terms of the levels of real interest rates, and this phase coincided with the great recession – not high growth, and (b) the second phase reflects coexistence of both high real rates and high growth. Thus, sustained ultra-loose monetary policy to deliver negative real rate as a means to spur growth is not always effective, and, high real rates that may result when a country moves from financial repression to reforms could actually deliver higher, not lower, growth. Besides financial repression/reforms, a central bank’s monetary policy response to inflation can also influence real interest rates, and thereby investment and growth.
Table 1: Real interest rates in advanced economies
(shares of observations at or below) |
Real interest rate |
1945-1980 |
1981-2007 |
2008-2011 |
<= 0 |
46.9 |
10.5 |
49.5 |
<= 1 per cent |
61.6 |
25.2 |
82.1 |
<= 2 per cent |
78.6 |
36.2 |
97.2 |
<= 3 per cent |
88.6 |
55.0 |
99.5 |
| Source: Reinhart, April 2012 (real ex-post Treasury Bill rates). |
Can monetary policy change real interest rates?
If investment is sensitive to changes in real rates, then for a central bank’s monetary policy to be effective, its changes in nominal rates should influence real rates. In other words, the direction of causation should be from nominal to real rates. Theoretical literature, however, suggests that real rate is a real phenomenon, and can be determined only by real factors. Given the real rate, depending on changing inflation expectations, nominal interest rate may change. Thus, the direction of causation is from inflation expectations to nominal rate, for a given real rate. The debate on nominal versus real rate, from the standpoint of role of monetary policy, however, offers different possibilities.
Henry Thornton (1802) was possibly the first one to differentiate between market (money) rate of interest on lonable funds and expected yield on new capital projects (or real rate). According to his assessment:(a) inflation could be the result of difference between money rate and real rate, and (b) inflationary expectations can create a wedge between the two. Thornton’s idea was reformulated, after about 100 years, by Knut Wicksell (1907)2, who also emphasised the difference between the “money rate (i)” and the “natural rate (R*)”. When these two rates are equal, desired savings (S) = desired investment (I), aggregate demand = aggregate supply, and hence there would be price stability. However, when i S, leading to credit/ money expansion, and hence inflation. What is particularly important to notice in this argument is that neither money is an exogenous determinant of inflation nor the causation runs from higher prices to higher money growth. Instead, both prices and money growth changes result in response to the difference between i and R*. Moreover, for the purpose of price stabilisation – knowledge of natural rate (R*) should not be a constraint. The simple rule should be – if prices are rising, raise the money rate (i). Unless money rate (i) is lower than the natural rate (R*), prices should not rise. Hence adoption of the simple rule should ensure price stabilisation.
The realised real rate is often presented in the form of an identity: r= i – p – r*p, where r is real rate, i is nominal rate and p is inflation (realised/expected). The cross-product part is often ignored at low inflation for policy analyses, but in a high inflation environment, the cross product could be significant. Irving Fisher (1936)3 used the same formulation, i.e. i = r+p+r*p, and also suggested limiting values (i.e. non-negative i), and most importantly, lagged adjustment of nominal rates to changes in inflation. Since nominal rates do not move exactly to offset price changes, realised (ex post) real rates do vary. That is, real rates are not constant, unlike Fama’s (1975) empirical emphasis on constant real rates. Since nominal rates do not respond one-for-one to changes in inflation: (a) realised real rates often move inversely in relation to nominal rates, (b) higher volatility is seen in real rates than in nominal rates, and (c) real rates often become negative during periods of high inflation (Mishkin, 1981 & 1984; Huizinga and Mishkin, 1986).
Thus, if monetary policy (i.e. higher nominal rate) responds to high inflation expectations – one for one - then constant real rate should materialise (i.e. current nominal rate would then reflect only market expectations of future inflation). However, if the response coefficient is less than one, real rate would change. In other words, lack of full adjustment of nominal rate to inflation is the driver of non-constant real rates. Summers (1982) suggested that both in the pre-war and post war-periods, there is little evidence of nominal rate rising one-for-one relative to increase in inflation. This he ascribed to possible money illusion in financial markets, i.e. agents used to possibly ignore inflation in financial calculations earlier, and over time, financial markets have become increasingly sensitive to inflation, but still not enough to remove money illusion completely. “...How unlikely is it that market participants should be unaware of the distinction between nominal and real interest rates? It is noteworthy that it was not until the 20th century that the distinction was even introduced into economic analysis. There is little evidence in mainstream economic writings in the l950's and 1960's of an awareness of this distinction.” Chadha and Dimsdale (1999) also found higher sensitivity of nominal rates to inflation in the post-war period, mainly after 1970s, which they ascribe to greater inflation focus of monetary policy; “...the lack of response of nominal interest rates to inflation in the early post-war years in all countries...can be explained by the high priority given to output relative to inflation”. With increasing focus of central banks on inflation, and several countries adopting explicit inflation targeting, the interest rate response to inflation has also increased (Table 2). According to Friedman and Schwartz (1976), financial markets are also learning to better conform to the Fisher effect. An obvious consequence of lack of one-for-one response of nominal rate to inflation is the negative relationship between observed real rate and inflation. When inflation rises, nominal rates may not increase as much (reflecting inflation tolerance of central banks), leading to lower real rates. Similarly, when inflation declines nominal rates may not decline as much, thereby leaving the real rates higher. The absence of Fisher effect, i.e. one-for-one change in nominal rate in response to change in inflation, is also evident in India (Table 3).
Table 2: Low-to-high Fisher Effects:
Regression Coefficients (short-term rates on inflation) |
Country |
Pre- WW II |
Post WW II |
1875-96 |
1897-1913 |
1920-38 |
1951-68 |
1969-79 |
1980-97 |
UK |
-0.02 |
0.10 |
0.00 |
-0.18 |
0.23 |
0.77 |
US |
0.08 |
0.22 |
-0.10 |
0.12 |
0.68 |
0.89 |
France |
0.01 |
0.01 |
0.05 |
0.03 |
0.60 |
0.69 |
Germany |
-0.04 |
0.01 |
0.02 |
0.29 |
0.74 |
0.96 |
Source: Chadha and Dimsdale (1999) |
Table 3: Weak evidence of Fisher Effect in India – a driver of non constant real rates? |
Time period |
variables |
Coefficient |
t-stat |
1997Q3 2012Q1 |
EFFECTIVE, WPIG |
0.62 |
1.74*** |
1998Q1 2012Q1 |
EFFECTIVE, WPIG |
0.49 |
1.72*** |
1998Q1 2012Q1 |
WPIG(-1), CALL |
0.44 |
2.14** |
2004Q2 2012Q1 |
WPIG(-1), CALL |
0.51 |
3.14* |
EFFECTIVE: Effective Policy Rate; WPIG: WPI Inflation (y-o-y); CALL: Weighted average call rate. All variables are stationary (results in Appendix Table 1)
*, **, ***: Significant at 1%, 5% and 10% level, respectively. |
When could a lower interest rate need not stimulate growth and investment?
It is a fact that weighted average lending rates in India since the 2008 global crisis have been lower than the rates that prevailed during the high growth phase before the global crisis (Section-IV). The obvious question that arises then is – if higher rates did not impact growth adversely during the high growth phase, why should a lower rate be blamed for the slowdown in growth since the global crisis? This could be explained by comparing the level of interest rate with the marginal productivity of capital at the macro level and internal rate of return (IRR) at the firm level.
At the macro-level, for policy purposes, two things are important: (a) sensitivity of investment to changes in interest rate, and (b) how the interest rate compares relative to changing marginal efficiency of capital. In terms of standard Keynesian analysis, the former could be explained through usual text book representation of the shape of the Marginal Efficiency of Investment (MEI) curve and the latter through the Marginal Efficiency of Capital (MEC) curve (Chart-1). The MEI curve shows sensitivity of investment to changes in interest rate. If the MEI curve is not very sensitive in a country, then larger cuts in rates could be desirable, if monetary policy has to bear the burden of reviving investment and growth. But in reality, this sensitivity may be time varying. Even with near zero policy rate and massive quantitative easing, advanced economies have not been able to revive durable growth. That is because of sharper fall in MEC, as reflected in continuous backward shifts in the MEC on account of depressed investment climate, intense deleveraging, and depressed return expectations on new investment. Even in India, reflecting the contagion from sluggish global growth and domestic governance concerns, the MEC would have shifted down, and this continuous downward drift cannot be prevented only with cuts in interest rate. For given MEC, by the time interest rate is lowered to incentivise investment, MEC may drop further, requiring a further cut in interest rate. In an environment characterised by continuously downward drifting MEC, repeated cuts in interest rate would amount to chasing a falling MEC, which at some point may become effective only if the factors driving the downward drift in MEC stop or reverse. If the factors causing the downward drift in MEC do not reverse, then repeated cuts in interest rate would amount to wasting the monetary policy space, and could become particularly dangerous in an environment characterised by persistently elevated risks to inflation.
 At the firm level, why a lower interest rate may not stimulate investment needs to be assessed by comparing the interest rate with internal rate of return (IRR). For a new investment project, what matters is the interest rate relative to IRR. If a particular amount of borrowed money (C) is used to acquire a capital good, which in turn is expected to yield a flow of return over say three years (of R1, R2 and R3), then the simple identity would yield the internal rate of return (r):
C = [R1/(1+r)] + [R2/(1+r)2] +[R3/(1+r)3]
The IRR is that rate of discount, which would equate discounted present value of expected return over three years to the cost of the project, making NPV (net present value) of the project equal to zero. If the interest rate [or the hurdle rate which is equal to the weighted average cost of capital (WAAC) plus risk premia]is lower than the IRR, the NPV of the project would be positive, thereby encouraging new investment. Thus, as long as interest rate is lower than IRR, additional investment will continue. In a period of economic slowdown, pro-growth monetary policy may push the interest rate down; but if the IRR also falls, and that too at a faster rate due to lower expected return on investment, then despite the support of a pro-growth monetary policy stance, IRR may remain below the interest rate, and thereby defeat the policy objective of promoting growth and investment through a lower interest rate. Thus, lower interest rate alone is not an indicator of pro-growth stance of policy. It should be seen relative to IRR. In a high growth phase, because of bullish expected return on investment, IRR may be higher, and therefore, a higher interest rate may still facilitate investment and growth. During a phase of economic slowdown, however, not only that the IRR falls, but the hurdle rate may also rise - despite lower policy interest rates – due to higher risk premium added to WAAC.
It is possible that, an increase in interest rate may also change the return prospects (i.e. R1, R2 and R3 may decline), which in turn will change (depress) the IRR. As a result, the pace of additional investment will slowdown. For the investment cycle, by how much return prospects change because of interest rate changes on the one hand and non-monetary factors on the other become important. For example, in an overheating phase of the business cycle, despite high interest rate, buoyant return prospects may prompt additional investment. Whereas in a slowdown phase, a lower interest rate alone may not improve return prospects, and need not trigger a fast recovery.
Extending such firm specific relationships to the level of the overall economy may create scope for ambiguities, particularly because investment horizons of different firms are different, and long-term interest rate expectations of different firms may be quite divergent. Using any single measure of interest rate to study the impact on investment demand, thus, would always have its own limitations. But monetary policy decisions have to be always based on macro-level interactions among key economic variables, and differential impact across sectors should not undermine the relevance of macro level relationships for conduct of policy. Notwithstanding the importance of sector specific polices to address sector specific concerns, for monetary policy, what matters is the transmission of policy rate changes to effective borrowing costs, and an average representative measure of effective cost of borrowing should provide useful evidence on the sensitivity of investment demand to interest rates in the economy. This paper, in Section-V, aims at estimating sensitivity of investment demand and GDP growth to changes in interest rate, using both aggregate macro-level data as well as sector level data.
In terms of simple explanation of the dilemma for monetary policy, it is important to recognise the distinction between movement along an investment demand curve, and shifts in the investment demand curve. A change in interest rate can at best help in influencing movement along the investment demand curve, given the degree of sensitivity (elasticity) of demand to changes in interest rates. The intended impact on investment demand may fail to materialise if non-monetary factors trigger a shift in the investment demand curve that works exactly in the opposition direction.
Which interest rate matters for investment – Real or Nominal?
While planning a new investment project, invariably it is the nominal interest rate which will go into project evaluation, but implicitly, inflation will also be part of the assessment of expected cash flows, which will be discounted to the present using the nominal interest rate to arrive at the net present value (NPV) of the project. Thus, even if explicitly a firm may not use real interest rate, implicitly it takes into account its own effective real interest rate, depending on its own inflation expectations as part of cash flow projections, and effective nominal cost of capital. For each firm, the duration of the project and hence the term period of borrowings as also the firm’s credit worthiness will together influence the firm specific borrowing costs (with firm specific term premium and risk premium) and the inflation expectations implicit in cash flows could also be quite different from other firms. For the economy as a whole, however, aggregate measures of effective cost of borrowings and inflation expectations could help in inferring the overall relationship between real cost of funds and investment trends. It is not that firms will behave similarly to similar levels of real rates at the macro level, when relevant nominal rates and inflation expectations are quite different across firms. For example a 3 per cent real rate could coexist with 5 per cent nominal rate and 2 per cent inflation and also 15 per cent nominal rate 12 per cent inflation. But high inflation itself may operate as an independent drag on investment, even if the real rate remains unchanged. This point comes to the fore clearly in the empirical estimates for India presented in Section-V.
Real interest rate – always a real phenomenon?
If monetary policy response to inflation can influence real rates, at least in the short-run, what happens to the argument then that real rate is a real – not a monetary phenomenon? Real rate changes should reflect thrift and productivity, the typical neo-classical view, that remains very much relevant in the long-run. Empirical literature also suggests that endogenously determined shifts in real interest rates could be explained by observed historical events, such as oil price shocks, large fiscal imbalances, stock market crash, and major tax reforms; “...it is still inconclusive whether monetary policy has had any effects on the real interest rate” (Manopimoke, 2008). Emphasising the role of nonmonetary factors in determining real rates, Allospp and Glyn (1999) noted that “...savings, population growth and technology determine the equilibrium, steady state, capital-to-output ratio and marginal product of capital, i.e. the interest rate.” Higher real rates, thus, could be the result of faster population growth, technological progress or lower savings.
Keynes (1936) was the first one to suggest explicitly that (nominal) interest rate is a monetary phenomenon, determined by the interaction between money demand and supply - contrary to the prevailing consensus till that time - and that the real economy should adjust to nominal interest rates, not the reverse.
As per the standard Keynesian analysis, interest rate is determined by the interaction of demand for money and supply of money. Exogenous money supply, which is determined by a central bank, will set the interest rate in the system for given money demand. Is the interest rate then a purely monetary phenomenon? The answer would be no, because even in the Keynesian system of macro-dynamics, the level of income is a determinant of interest rate through money demand, which in turn is also influenced by the interest rate. Interest rate relative to marginal efficiency of capital influences the investment demand, and given the multiplier - for given propensity to save - income level is influenced. The dynamic interaction, thus, suggests that interest rate may be determined by the interactions between money demand and money supply, but interest rate itself can influence money demand, through the influence on investment demand.
Oscar Lange’s representation of the same dynamics assumes somewhat more realistic possibilities. Unlike Keynes, consumption is viewed as a function of both income and interest rate, and investment a function of both consumption demand and interest rate. Since both consumption and investment demand could be partly credit financed, and because that consumption demand can influence investment demand, this specification has been used more often in empirical research on the subject.
The Keynesian prescription had to face stiff resistance from the super-neutrality proposition, that suggested neutrality of money- or inability of money to influence real variables - in both short-run and long-run. In the subsequent period, a third view on interest rate evolved (the classical lonabale funds theory and Keynes’ liquidity preference theory being the first two), which is the horizontalist view propounded by Moore (1988). According to this, money is endogenous (unlike Keynes’s exogenous money), and nominal interest rate is exogenously determined by the central bank. For given interest rate, money supply will change along the horizontal line, representing full accommodation of money demand by the central bank. There was a fourth view also, as suggested by Wray (1992), according to which both interest rate and money supply are determined endogenously. This view reflects the possibility that the money supply curve may be upward sloping, implying that banks will increase the money supply when demand for money increases, but will expect higher interest rate to supply higher money. Thus, depending on whether money supply is vertical (Keynes’s view), horizontal (Moore’s view) or upward sloping (Wray’s view), the determination of interest rate as a monetary factor will vary. According to Smithin (2003), Moore’s endogenous money supply and exogenously determined policy rate is the contemporary institutional reality and “...if the natural rate concept is dubious, it seems clear that central bank power to control nominal short term rates of interest should also be thought of as power to control the real rate also”. Given the fact that central banks determine policy rates, influence the term structure of interest rates, and also the fact that they change policy rates based on assessment of inflation expectations for the economy as a whole, it would be only proper to infer that monetary policy influences real rates. Moreover, reference to real rate trends for assessment of the effectiveness of monetary policy has also increased over time in central bank publications. Upper and Worms (2009), based on their cross-country estimates, concluded that “...both monetary and fiscal policy generally play an important role in the determination of long-term real interest rates”.
The above debate suggests that real interest rate can be influenced by monetary policy, but the underlying drivers of real interest rate will most likely be dominated by factors that influence real savings and real investment. These factors could include fiscal deficit, corporate tax, stock market return, commodity prices, inflation, technological progress, and business confidence. For example, if the fiscal deficit is lower, that will increase the saving rate, and thereby depress real rates. Higher expected profitability on investment - when the stock market is bullish - could raise real rates. Shocks to income, say through higher oil prices, could depress domestic savings, and thereby raise real rates. In essence, if domestic saving is less than domestic investment, real rates could be high, unless inflows of foreign savings close the domestic savings investment gap. Saving-investment gap and productivity of investment, thus, are the key determinants of real interest rate.
The overall policy relevant inference from this section is that both real and monetary factors influence real rates in real life. Non-constant real rates - reflecting monetary policy response being the key driver of deviations from the fisher effect - may have to be seen as a key justification for real rate not being a real phenomenon all the time. Unlike the exogenous money supply argument of Keynes, central banks generally announce the nominal interest rate and manage liquidity to keep the near term rates anchored to policy rates. The demand for liquidity is accommodated automatically, to avoid large deviations of market interest rates from policy rate. As per this endogenous money supply view, thus, credit and money growth should be entirely demand driven, and a central bank has to passively accommodate the changing demand by ensuring liquidity conditions that help anchor the market rates to policy rates. The only way a central bank could influence money and credit growth is by changing the policy interest rate, and it is the change in interest rate which influences money and credit demand. Thus, central banks critically depend on monetary policy transmission to influence aggregate demand, including investment demand. A lower policy rate - if it can transmit to lending rates and if demand for credit is sensitive enough to lower rates - then monetary policy can stimulate investment demand.
Section-III: A brief review of empirical research on the impact of changes in
real interest rate on investment and growth
Empirical research suggests three broad possibilities on the relationship between real interest rate and economic growth: (a) higher real interest rates leading to higher growth – the “financial repression to reform” view of McKinnon and Shaw (1973), (b) higher real rates leading to lower growth – the standard “monetary policy” view, and (c) higher real rates leading to higher or lower growth depending on the level of the real rate relative to the threshold level - i.e. the non-linear relationship highlighted by Fry (1997). Pill and Pradhan (1995) noted that the McKinnon and Shaw relationship may not hold if the capital account of a country is open. In other words, high growth could co-exist with both modest real rates and an open capital account.
Financial sector liberalisation could end financial repression and thereby lead to end of negative or low real interest rates. But responding to more attractive real returns, domestic saving rate could increase, and because of higher expected return on new investments in a liberalised environment, investment may also increase, despite higher real rates. Pill (1997) found that negative real interest rates of 25 per cent could be associated with stagnation of real per-capita income, while with financial liberalisation, the rise in real to the positive territory of about 5 per cent, could raise real per capita GDP by 2 per cent per annum. In another cross-country study covering 101 developing countries for the period 1970-2007, Mehrara and Karsalari (2011) highlighted that private investment is negatively impacted by real rates beyond the threshold level of 5 to 6 per cent, but within the threshold level, real rates exhibited a positive impact on private investment. The inverted U shaped relationship between real interest rate and private investment does not rule out the possibility that lower real rates are congenial to growth while very high real rates are harmful to growth.
According to one G-10 study (1995), 100 bps increase in real interest rates to about 4 per cent in G-10 countries over a 35 year period since 1960s coincided with decline in saving rate by about 5 per cent of GDP to below 20 per cent, which was more pronounced than the moderation in investment rate. This high real interest rate period, nevertheless, coincided with lower investment rate, slowdown in growth of total factor productivity, and decline in measured rates of return on capital, even though the study did not ascribe all these outcomes to higher real rate. Taylor (1999), reviewing the empirical literature on the relationship, underscored the mixed evidence on the existence of a positive relationship between real rate and growth.
In China, according to estimates provided by Geng and N’Diaye (2012), 100 bps increase in real rate lowers corporate investment by 0.5 per cent of GDP. Since real rates are much lower than the marginal productivity of capital, which in turn is a source of investment driven macro-imbalance, they suggested higher real rates closer to MPC to rebalance the economy, with lower investment and more consumption.
In India, Mallick and Agarwal (2007) found that none of the three measures of real interest rate (call rate, 91 T Bill rate, and 364 T Bill rate) seemed to exert any direct influence on growth of real output. This unusual result they ascribed to the possibility that investment, which is an important determinant of growth, is conditioned by several factors other than real interest rate alone. Mohanty, Chakraborty and Gangadaran (2012), on the other hand, highlighted the presence of inverse relationship between growth and real lending rates in India, with empirical evidence on real lending rates Granger causing both overall GDP and non-agricultural GDP growth. Tokuoka (2012) found evidence of negative impact of increase in real interest rate on corporate investment in the macroeconomic data (with the impact ranging between 51 to 34 bps in different estimates for 100 bps change in real interest rate), while for the firm level data profitability, liquidity and leverage were highlighted as the key determinants of corporate investment in India. IMF (2013) estimates highlighted that compared with nominal interest rate the real interest rate explains better fluctuations in economy wide investment activities, and also suggested that one fourth of the explained slowdown in investment activities could be attributed to high real interest rates.
The review of empirical literature, thus, does not offer any unambiguous guidance on how real interest rate should be seen by policy makers, while trying to influence growth and investment for stabilising the business cycle. For central banks, however, the effectiveness of normal monetary policy transmission depends largely on the ability of monetary policy actions – in terms changes in policy rates, quantity of liquidity and forward guidance – to influence deposit and lending rates, which in turn should influence saving, investment and growth. Country specific assessment of the relationship between real interest rate and growth is relevant not only from the stand point of assessing the last major leg of policy transmission, but also for recognising the role of non-monetary factors in conditioning the path of real interest rates, investment and growth. The empirical estimates presented in Section-V focus only on the former, consistent with the scope of this paper.
Section-IV: Policy inferences from firm-level and macroeconomic data
Policy relevant inferences could often be derived from recent trends in relevant data, and this section aims at that. Firm level data for a common set of about 830 firms over the period 2000-01 to 2010-11 indicate that interest costs as percentage of total expenditure averaged at only about 3.2 per cent in the last decade, which fell to 2.2 per cent in 2010-11 (Chart-2). Moreover, the shares of interest cost in total sales and total expenditure have declined over time in last one decade (Chart-3). This fact in itself may suggest that real or nominal interest rate does not matter much for investment and growth, but when seen relative to MPC (at the macro level) and hurdle rate (at the firm level), as highlighted in Section-II, the impact could be significant.
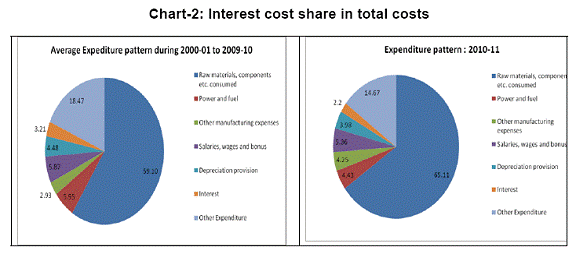
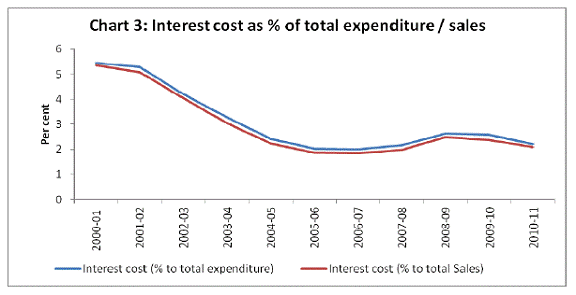 For the corporate sector, estimate of effective cost of borrowings is proxied by annual interest payment as ratio of outstanding average borrowing. As depicted in Chart-3, effective nominal borrowing costs of corporates have declined over time, to about 6 per cent. The advantage of using average of outstanding borrowings of previous year and current year is to capture the impact of interest rate changes during the current year, though new borrowings and interest payments on those borrowings are not available separately. However, the limitation of using this data for empirical estimation could be that the estimated effective cost captures the impact of costs of both domestic and external debt, since they are not available separately. As one would expect, the nominal effective cost of borrowings varies across 24 sectors, as also interest costs as percentage of total expenditure, pointing to likely differential degree of sensitivity of sectors to changes in interest rates (Chart- 5 and 6).


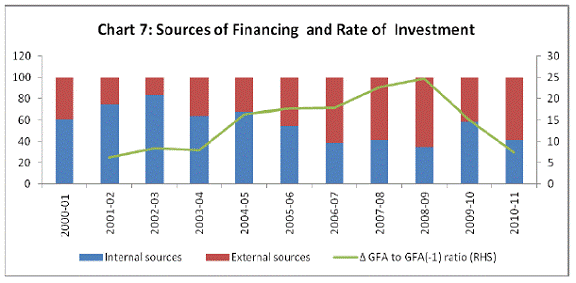
 For the firm level data, a proxy for investment could be change in fixed assets. Rate of growth in fixed assets exhibits similar trend as growth in gross fixed capital formation (GFCF) from the expenditure side GDP data. It could be seen from Chart- 7 that investment demand decelarated sharply in last two years (a fact corroborated by the sharp decleration in growth of GFCF), and this happned despite lower effective borrowing costs (Chart-4). It is important, therefore, to recognise the role of falling marginal productivity of capital and hurdle rate for explaining the negative relationship between real or nominal interest rate and investment demand.
 In recent quarters, both GFCF growth and GDP growth have decelerated. Since quarterly data on capital stock are not available, GFCF is taken as a proxy for change in capital stock. GFCF as a percentage of expenditure side GDP would be equivalent of investment rate. Investment rate as a ratio of growth in supply side GDP could provide an indicative trend ICOR (incremental capital output ratio), which appears to be rising over last several quarters. Marginal productivity of capital (MPC), which is [1/ICOR] correspondingly has gradually falling over successive recent quarters. It is important to compare the declining MPC against recent trends in nominal and real lending rates (Chart 8).
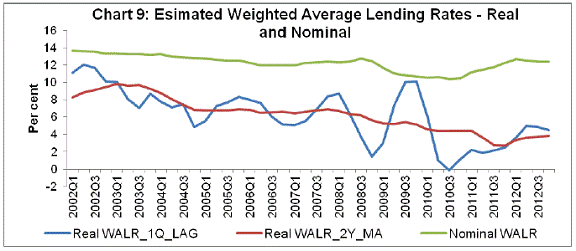
 Estimation of real interest rates requires information on both nominal interest rates and relevant measures of inflation expectations. While some studies extract inflation expecations either from inflation expectations surveys or yields on inflation indexed bonds in the secondray market, given the limitations of such approximations in emerging economies, it is a common practice to use average inflation of the recent past, which though would be a backward-looking measure of expectations for the future. In this paper, averge inflation during the immediately preceding quarter, and immediately preceding last two years have ben used. The weighted average annual nominal lending rates based on Basic Statistical Returns of scheduled commercial banks are published in Mohanty et al. (2011). From the annual data, quarterly weighted average nominal rates were estimated using the interest rate movement in the “yields of AAA rated bonds issued by Indian corporate with 5-year maturity”.Two sets of real average lending rates were estimated. The 2 year moving weighted average real lending rate ehxibits a declining trend in recent quarters. (Chart- 9) When this real rate is compared with derived MPC, it is clear that despite lower real rates, because of the falling MPC, new investment may not be attractive. This provides a factual justification as to why monetray policy is not the only factor behind the slowdown in growth and investment in recent quarters.
Section-V: Empirical Evaluation
Both nominal and real interest rates may interact with the ultimate goal variables of macroeconomic policy - such as growth, investment and inflation - in a complex way and no single statistical methodology may be adequate to provide precise impact estimates for policy making. The preferred option then could be to use an array of methodologies, applied to both macro-level and sector-level data available for a country, so that some broad policy relevant empirical inferences could be derived. In the same spirit, this paper uses different methodologies and data sets for the empirical testing of the hypothesis relating to the impact of changes in interest rate on growth and investment. One needs to recognize that this at best is only one part, but an important part, of the monetary policy transmission analysis. How monetary policy changes impact the lending rates falls outside the scope of this paper. Many papers on monetary policy transmission try to comprehensively cover all transmission steps, but this paper focuses on the transmission from lending rates to real activity. Changes in real lending rates could reflect both monetary and non-monetary factors, and this paper is not about determinants of real interest rate. Empirical estimates in this paper focus primarily on whether investment demand and overall growth are sensitive to changes in real and nominal lending rates, and if so, how much.
Panel Regression Results
Panel fixed effect models (FEM) are used throughout the study as they were preferred over random effect models (REM), validated by both Hausman test and Redundant Fixed Effect test. Generalized Least Square (GLS) estimation was preferred over ordinary least square (OLS) to correct for heteroscedasticty. The standard errors are corrected for autocorrelation and heteroscedasticity and robust coefficient covariances are achieved using panel corrected standard errors (PCSE) with degrees of freedom adjusted, as proposed by Beck and Katz (1995). To get sector specific interest rate sensitivity effects, the estimation process allows interaction of COST with sector specific dummies in the FEM. Apart from these, Quantile regression is also used to examine the heterogenous impact of interest rates changes on investment/profits at various quantiles.
Impact of increase in nominal effective cost of funds on investment
Estimated results (reported in Table 4) suggest that for a 100 bps increase in the nominal cost of debt funds, investment (i.e. growth in fixed assets) could decline in the range of 62 to 91 basis points. Of the four different panel estimates presented in Table-4, the dynamic panel estimates4 point to 68 bps decline in growth of fixed assets (GFAC) in the short-run and 91 bps in the long-run for 100 bps increase in interest rate. GLS-fixed effect estimates yield an impact of 87 basis points. Dynamic panel estimates with leverage as an additional variable (proxied by debt/equity ratio and debt to total assets ratio) indicate that the short-run impact could be about 48/54 bps, and long-run impact about 62/70 bps. The impact, however, varies for different sectors, with some sectors showing significant sensitivity to interest rate changes while others do not show any statistically significant sensitivity (Table 5). These sector specific estimates relate to overall GLS-fixed effect estimates presented in the third column of Table-4. The differential sensitivity across sectors reflects differences in the relative shares of interest expenses in total costs and also the pace of growth in fixed assets across sectors over time. The divergence in estimated sensitivity is also cross-checked through quantile regression (reported later). When real variables (real effective cost, real GFA growth, real sales growth) are used in the place of nominal variables in FEM, the impact is somewhat lower (reported later).
Table-4: Estimated Impact of Cost on Investment (Dependent Variable: GFAG) |
|
FEM-GLS |
Dynamic-GMM |
Dynamic-GMM
(Debt-Asset) |
Dynamic-GMM
(Debt-Equity) |
|
Coeff. |
t-Stat |
Coeff. |
t-Stat |
Coeff. |
t-Stat |
Coeff. |
t-Stat |
GFAG(-1) |
|
|
0.26 |
89.02* |
0.23 |
31.89* |
0.23 |
42.08* |
COST(-1) |
-0.87 |
-5.84* |
-0.68 |
-35.98* |
-0.54 |
-12.19* |
-0.48 |
-17.59* |
SALG(-1) |
0.15 |
4.21* |
0.04 |
5.87* |
0.07 |
3.55* |
0.05 |
4.32* |
LEVG(-1) |
|
|
|
|
-0.42 |
-27.26* |
-0.06 |
-41.79* |
Long-Run
Coeff. of GFAG |
|
|
-0.91 |
|
-0.70 |
|
-0.62 |
|
R2 |
0.35 |
|
|
|
|
|
|
|
DW |
1.78 |
|
|
|
|
|
|
|
J-statistics |
|
|
21.91 (0.41) |
21.45 (0.43) |
23.53 (0.32) |
No. of Observations |
264 |
|
216 |
|
216 |
|
216 |
|
Note: Figure in parenthesis is p-value for J-stat.
Instrument variables are lagged values of variables used in the equation
White standard errors & covariance (d.f. corrected)
*: Significant at 1% level. |
Table 5: Estimates of sector specific investment sensitivity to changes in Interest Rates
(Dependent Variable: GFAG) |
Variable |
Coefficient |
t-Statistic |
P-value |
C |
19.01* |
7.93 |
0.00 |
Food Products & Beverages |
-2.65* |
-2.61 |
0.01 |
Sugar |
-4.26* |
-2.61 |
0.01 |
Cotton Textiles |
-2.06* |
-2.27 |
0.02 |
Man-made Textiles |
-1.32* |
-2.07 |
0.04 |
Paper Products |
-0.04 |
-0.03 |
0.97 |
Chemical Products |
-1.13** |
-1.93 |
0.05 |
Basic Chemicals |
-1.31* |
-4.38 |
0.00 |
Fertilisers |
-1.17 |
-0.90 |
0.37 |
Paints & Varnishes |
-0.89 |
-1.10 |
0.27 |
Pharmaceuticals |
0.09 |
0.18 |
0.86 |
Rubber & Plastic Products |
-1.50* |
-3.57 |
0.00 |
Plastic Products |
-2.43 |
-1.32 |
0.19 |
Cement & Cement Products |
-2.48* |
-3.99 |
0.00 |
Iron & steel |
-2.89* |
-2.73 |
0.01 |
Fabricated metal Products |
-0.67 |
-1.02 |
0.31 |
Machinery & accounting |
-0.90* |
-2.36 |
0.02 |
Electrical machinery |
0.07 |
0.08 |
0.94 |
Radio TV communication equip |
1.17* |
2.09 |
0.04 |
Motor vehicles & transport |
-1.44* |
-3.37 |
0.00 |
Construction |
-2.11 |
-0.91 |
0.36 |
Trade wholesale & retail |
4.21* |
2.89 |
0.00 |
Hotel & restaurants |
1.27 |
1.46 |
0.15 |
Transport storage |
-4.94** |
-1.88 |
0.06 |
Computer |
4.15* |
2.90 |
0.00 |
SALG(-1) |
0.09 |
2.57 |
0.01 |
R2 |
0.46 |
DW |
2.06 |
*, **: Significant at 5% and 10% level, respectively. |
(i) Sensitivity of profitability to changes in nominal effective cost of funds
Profitability could be a more proximate determinant of investment, and interest rate may impact investment by altering profitability. To explore this possibility, panel GLS regression of PBITSAL on COST and SALG was estimated. Results point to 25 bps erosion in profitability for 100 bps increase in costs (Table 6).
(ii) Impact of increase in real effective cost of funds on investment.
Given the fact that inflation expectations of each firm could be different from others, and also that aggregated inflation expectations of each sector could be different from other sectors, arriving at real effective cost of funds for 24 sectors from the derived data on nominal effective cost of funds is an empirical challenge. In the absence of sectoral data on inflation matching the sectoral classification used from firm level data in this paper, a common measure of inflation expectation (i.e. average inflation of last year) has been used as the deflator for all 24 sectors. GLS estimates of FEM point to statistically significant sensitivity of investment to real interest rate changes (Table 7). For 100 bps increase in real effective borrowing costs, investment declines by 55 basis points.
Table 6: GLS estimates of Fixed Effect Model (Dependent Variable: PBITSAL) |
|
Coefficient |
t-Statistic |
C |
14.33* |
30.60 |
SALG |
0.05* |
4.13 |
COST |
-0.25* |
-5.55 |
R2 |
0.81 |
|
DW |
1.29 |
|
Hausman χ2 |
29.77 (0.00)* |
|
Redundant Fixed effect F-stat |
45.55 (0.00)* |
|
No. of Observations |
288 |
|
Note:t-statistics are based on cross-section weights (PCSE) standard errors &
covariance
(d.f. corrected)
Figures in parantheses are p-values.
*: Significant at 5% level. |
Table 7: GLS estimates of Fixed Effect Model (Dependent Variable: RGFAG) |
|
Coefficient |
t-Statistic |
C |
7.92* |
11.52 |
RCOST(-1) |
-0.55* |
-5.10 |
RSALG(-1) |
0.15* |
4.14 |
R2 |
0.32 |
|
DW |
1.70 |
|
Hausman χ2 |
29.00 (0.00)* |
|
Redundant Fixed effect F-stat |
2.83(0.00)* |
|
No. of Observations |
264 |
|
Note: t-statistics are based on cross-section weights (PCSE) standard errors & covariance (d.f. corrected)
Figures in parantheses are p-values.
*: Significant at 5% level. |
Quantile Regression Results
While the panel regression results help in estimating the mean relationship between the explained variable and the explanatory variables, there could be significant diversity around the mean. For example, the interest rate sensitivity of different sectors could be different, and the sensitivity could also vary over time. Quantile regression helps in presenting the change in the relationship over different quantiles. Particularly, the quantile regression parameter estimates the change in a specified quantile of the response variable produced by a one-unit change in the independent variable. As could be seen from Table 8, as against the mean relationship suggesting 76 bps change in investment per 100 bps change in nominal cost of debt funds, the median impact is about 88 bps (for the 50th quantile or 5th decile), and the statistically significant relationship holds for the intermediate deciles, and the sensitivity also increases gradually. In extreme quantiles, the relationships are not statistically significant (Chart-10). The coefficient value also varies from 0.29 to 1.05 at different quantiles. Qunatile regression using real effective cost of funds (RCOST) and real investment (GFAG) and real sales growth (RSALG) shows a median sensitivity of 60 bps in response to a 100 bps change in real cost of borrowings (Table 9).
Table 8: Comparison of Quantile estimates with FEM estimates (GFAG) |
|
GLS -FEM |
Quantile Regression Estimates |
0.1 |
0.2 |
0.3 |
0.4 |
0.5 |
0.6 |
0.7 |
0.8 |
0.9 |
Constant |
18.65 |
4.57 |
9.56 |
10.63 |
13.61 |
15.40 |
18.66 |
20.32 |
22.41 |
26.75 |
t-stat |
(11.31)* |
(1.38) |
(3.56)* |
(5.58)* |
(7.44)* |
(8.83)* |
(9.49)* |
(9.17)* |
(8.26)* |
(4.64)* |
COST(-1) |
-0.87 |
-0.29 |
-0.61 |
-0.62 |
-0.79 |
-0.84 |
-1.02 |
-1.05 |
-1.05 |
-0.99 |
t-stat |
(-5.84)* |
(-1.10) |
(-2.90)* |
(-3.65)* |
(-4.66)* |
(-5.05)* |
(-5.50)* |
(-4.86)* |
(-3.68)* |
(-1.39) |
SALG(-1) |
0.15 |
0.10 |
0.17 |
0.21 |
0.20 |
0.20 |
0.23 |
0.29 |
0.37 |
0.43 |
t-stat |
(4.21)* |
(1.34) |
(2.36)* |
(7.56)* |
(6.93)* |
(6.85)* |
(4.88)* |
(4.34)* |
(5.77)* |
(4.16)* |
*, **: Significant at 5% level. |
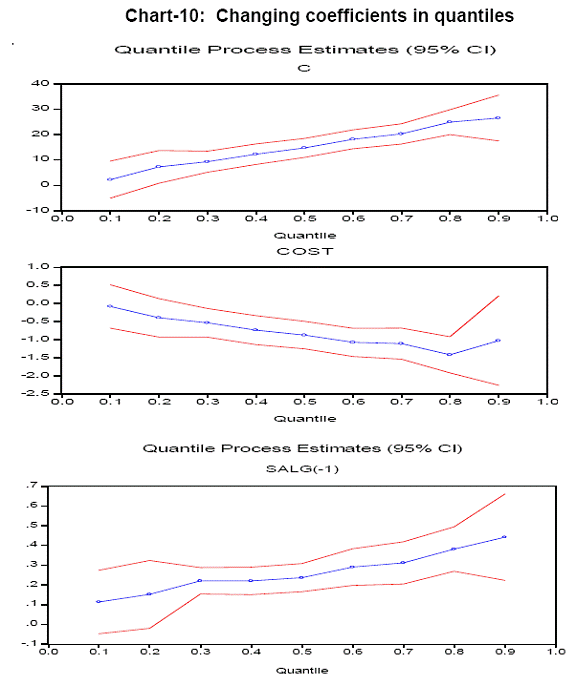
Table 9: Comparison of Quantile estimates with FEM estimates (RGFAG) |
| |
GLS-FEM |
Quantile Regression Estimates |
0.1 |
0.2 |
0.3 |
0.4 |
0.5 |
0.6 |
0.7 |
0.8 |
0.9 |
Constant |
7.92 |
-2.91 |
0.25 |
2.48 |
4.04 |
5.75 |
7.65 |
9.63 |
12.42 |
17.27 |
t-stat |
(11.52)* |
(-1.97)** |
(0.27) |
(3.05)* |
(4.91)* |
(6.59)* |
(7.59)* |
(7.75)* |
(8.50)* |
(9.67)* |
RCOST(-1) |
-0.55 |
-0.14 |
-0.37 |
-0.46 |
-0.52 |
-0.60 |
-0.73 |
-0.71 |
-0.78 |
-0.78 |
t-stat |
(-5.10)* |
(-0.77) |
(-2.79)* |
(-3.87)* |
(-4.20)* |
(-4.47)* |
(-4.83)* |
(-3.64)* |
(-3.13)* |
(-1.57) |
RSALG(-1) |
0.15 |
0.14 |
0.19 |
0.18 |
0.18 |
0.20 |
0.21 |
0.23 |
0.36 |
0.49 |
t-stat |
(4.14)* |
(1.92) ** |
(4.83)* |
(4.77)* |
(5.42)* |
(5.70)* |
(4.96)* |
(3.59)* |
(4.96)* |
(5.57)* |
*, **: Significant at 5% and 10% level, respectively. |
OLS Estimation using Macro-level data
Since the impact of monetary policy changes on investment and growth assumes particular significance at the macro-economic level, sensitivity of investment, in terms of both investment to GDP ratio (IGDP) and growth in gross fixed capital formation(GFCF) to changes in real effective lending rate has been estimated using OLS, along with other determinants of investment. Unit root test results of the variables used in the study are presented in Appendix Table 1, which suggest that all variables are I(0).
(i) Impact of 100 bps increase in real effective lending rate on GDP growth.
GDP growth (GDPG) is regressed on real lending rate (i.e. nominal lending rate minus average inflation of one quarter lag to capture inflation expectations, WALR1QLAG) and other determinants such as world GDP growth (WGDP), fiscal deficit to GDP (GFD) with lags, and inflation exceeding a threshold of 6 per cent5 (INFLTR) and the results are reported in Table 10. Estimates point to 19 bps reduction in GDP growth for 100 bps increase in the real lending rate in the short run. Two striking findings that merit particular attention is the negative impact of GFD and INFLTR on growth. If lower real lending rate is attained through higher inflation tolerance and a pro-growth policy rate response, then higher inflation could erode growth more than the extent to which lower real rate can improve growth. Moreover, unlike the Keynesian prescription favouring fiscal stimulus for promoting growth, higher fiscal deficit adversely impacts growth generally, with lags.
Table 10: OLS estimates of GDP Growth (GDPG) |
|
Coefficient |
t-Statistic |
C |
4.23 |
2.18* |
WGDP |
0.20 |
1.75** |
GFD(-3) |
-0.13 |
-2.99* |
WALR1QLAG(-1) |
-0.19 |
-1.9** |
GDPG(-1) |
0.61 |
3.14* |
INFLTR |
-0.27 |
-3.35* |
D2003Q4 |
2.89 |
8.50* |
D2009Q1 |
-2.92 |
-4.37* |
D2010Q1 |
5.79 |
8.21* |
R2 |
0.63 |
|
DW |
1.85 |
|
SE of regression |
1.39 |
|
No. of Observations |
43 |
|
Note: t-statistics are based on HAC standard errors corrected with Newey-West/Bartlett window and 3 lags.
*: Significant at 5% level. |
(ii) Impact of 100 bps increase in real effective lending rate on non-agricultural GDP growth (NAG).
Estimated coefficients presented in Table 11 indicate weaker impact of increase in real interest rate on growth in non-agricultural GDP relative to overall GDP growth. The short-run sacrifice of growth for 100 bps increase in real lending rates comes to about 9 bps, and the long-run impact is higher at about 20 bps. The significance of INFLTR relative to real rate in influencing non-agricultural GDP growth is equally stark as in the case with overall GDP growth.
Table 11: OLS estimates of Non-agricultural GDP Growth (NAG) |
|
Coefficient |
t-Statistic |
C |
3.77 |
2.14* |
NAG(-1) |
0.54 |
3.27* |
WGDP |
0.18 |
4.40* |
WALR1QLAG(-1) |
-0.09 |
-2.35* |
INFLTR(-1) |
-0.12 |
-2.26* |
D(NFCGDP_SA(-4)) |
0.09 |
3.80* |
D2009Q3 |
2.45 |
18.50* |
R2 |
0.61 |
|
DW |
2.08 |
|
SE of regression |
0.93 |
|
No. of Observations |
40 |
|
Note: t-statistics are based on HAC standard errors corrected with Newey-West/Bartlett window and 3 lags.
*: Significant at 5% level. |
(iii) Impact of 100 bps increase in real lending rate on investment.
Given the perception in last more than one year that RBI’s monetary policy contributed to the investment slowdown, two alternative measures of investment were used in the estimation, i.e. investment to GDP ratio - seasonally adjusted (IGDP) and rate of growth in gross fixed capital formation (GFCFG). The results show that a 100 bps increase in real lending rate lowers investment to GDP ratio by 9 bps in the short-run and 51 bps in the long-run (Table 12) and lowers GFCFG by 46 bps in the short-run and 55 bps in the long-run (Table 13). The adverse impact of inflation exceeding a threshold level of 6 per cent on investment is also negative (Table 12), as was the case with GDP growth, but the notable point here is that the INFLTR impact is weaker than real lending rate. Thus, accommodative monetary policy that raises inflation tolerance to lower real rate can improve investment rate, but higher inflation would still lower overall GDP growth, possibly by depressing real consumption demand. If inflation persists at high level, its moderating impact on consumption demand would lower growth, even when lower real lending rate may be favourable to investment demand. Estimates presented in Table 14 validate this point that when private consumption demand is included as an additional determinant of investment, lower consumption demand adversely impacts investment rate.
Table 12: OLS estimates of Investment Rate
(Dependent Variable: IGDP) |
|
Coefficient |
t-Statistic |
C |
4.40 |
4.97* |
GDPG(-1) |
0.15 |
2.85* |
WALR1QLAG(-1) |
-0.09 |
-3.46* |
INFLTR |
-0.04 |
-1.88** |
IGDP(-1) |
0.83 |
25.93* |
DCRISIS |
-1.95 |
-7.18* |
∆NFCGDP(-4) |
0.09 |
4.33* |
R2 |
0.94 |
|
DW |
2.27 |
|
SE of regression |
0.73 |
|
No. of Observations |
40 |
|
Note: t-statistics are based on HAC standard errors corrected with Newey-West/Bartlett window and 3 lags.
*, **: Significant at 5% and 10% level, respectively. |
Table 13: OLS estimates of Investment Growth (GFCFG) |
|
Coefficient |
t-Statistic |
C |
5.00 |
0.87 |
GFCFG(-3) |
0.17 |
4.30* |
GDPG(-1) |
0.76 |
1.81** |
WALR1QLAG(-3) |
-0.46 |
-1.68** |
D2010Q1 |
26.29 |
22.16* |
D2009Q1 |
-12.34 |
-4.42* |
AR(1) |
0.83 |
9.00* |
R2 |
0.78 |
|
DW |
1.98 |
|
SE of regression |
3.82 |
|
No. of Observations |
37 |
|
Note: t-statistics are based on HAC standard errors corrected with Newey-West/Bartlett window and 3 lags.
*, **: Significant at 5% and 10% level, respectively. |
(iv) Impact of 100 bps increase in nominal lending rate on investment
Even though investment, as a real variable, is expected to be influenced by real lending rate, in real life much of the pricing and investment decisions are taken and planned in nominal terms. It could be interesting, therefore, statistically, to also assess the sensitivity of real investment to changes in nominal lending rates. Table 15 estimates suggest that for 100 bps increase in nominal lending rate, investment rate could decline by 11 bps in the short run and 61 bps in the long run.
Table 14: OLS estimates of Investment Rate (IGDP) |
|
Coefficient |
t-Statistic |
C |
5.35 |
4.24* |
PFCE(-1) |
0.14 |
2.28* |
WALR1QLAG(-1) |
-0.09 |
-3.67* |
INFLTR |
0.00 |
-0.20 |
IGDP(-1) |
0.80 |
16.33* |
DCRISIS |
-1.97 |
-6.23* |
∆NFCGDP(-4) |
0.11 |
3.19* |
R2 |
0.94 |
|
DW |
2.06 |
|
SE of regression |
0.74 |
|
No. of Observations |
40 |
|
Note: t-statistics are based on HAC standard errors corrected
with Newey-West/Bartlett window and 3 lags.
*: Significant at 5% level. |
Table 15: OLS estimates of Investment Rate (IGDP) |
|
Coefficient |
t-Statistic |
C |
5.31 |
8.11* |
GDPG(-1) |
0.17 |
3.12* |
WALR(-1) |
-0.11 |
-2.98* |
INFLTR(-1) |
0.07 |
5.25* |
IGDP(-1) |
0.82 |
33.67* |
DCRISIS |
-1.99 |
-12.95* |
∆NFCGDP(-4) |
0.09 |
6.09* |
R2 |
0.94 |
|
DW |
2.24 |
|
SE of regression |
0.72 |
|
No. of Observations |
40 |
|
Note: t-statistics are based on HAC standard errors corrected with Newey-West/Bartlett window and 3 lags.
*: Significant at 5% level. |
Vector Auto Regression (VAR) Estimates
The dynamic interactions between real interest rate, growth and inflation could be complex, and there is obvious endogenity among these three variables. Hence, deciding a priori real lending rate as a determinant of growth and inflation may fail to capture the dynamics arising from endogenity. To account for this possibility, a structural VAR was used, and the impulse response coefficients validated the expected negative impact of increase in real lending rate on both growth and inflation (Chart-11). Cumulative impulse response over successive quarters indicate that for a shock to real interest rate (equivalent of 25 bps increase in the real rate), non-agricultural GDP growth declines by 7 bps. In other words, for 100 bps increase in real lending rate, non-agricultural GDP growth could decline by about 28 bps, which is close to 20 bps long-term impact estimated through OLS, as presented above.
Impact of higher interest rate on components of aggregate demand
Of the three major domestic components of expenditure side GDP, it is generally perceived that government expenditure is not sensitive to interest rate changes, and even private consumption demand, due to low leverage, is also not very sensitive. Thus, any higher interest rate policy to contain aggregate demand and thereby contain inflation may operate primarily by depressing investment demand. Given the statistically significant adverse impact of both higher nominal and real lending rates on investment demand as estimated above, the impact on private final consumption expenditure (PFCE) and government final consumption expenditure (GFCE) were also estimated.
Private final consumption expenditure in India, unlike in advanced economies, is not much dependent on availability of credit as yet, even though access to personal loans and consumption related credit has improved over the years to facilitate consumption smoothing. As could be seen from Chart-12, consumption credit (i.e. personal loans) as percentage of PFCE and total credit have declined over time. Accordingly, PFCEG does not exhibit statistically significant sensitivity to changes in nominal lending rates, though the impact of real lending rates on growth in private consumption is weakly significant (Table 16, 17a and 17b).
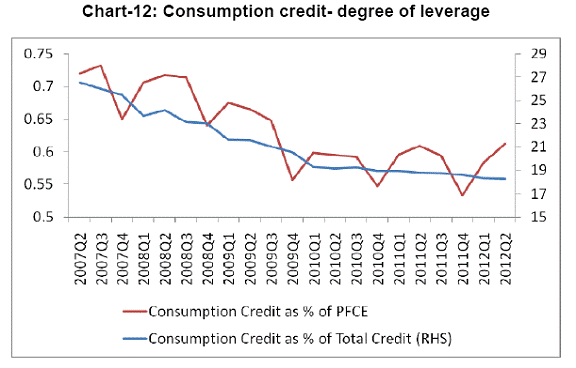
Table 16: OLS estimates of impact of WALR on Private Consumption Growth (PFCEG) |
|
Coefficient |
t-Statistic |
C |
7.64 |
1.35 |
WALR(-1) |
-0.36 |
-0.83 |
PFCEG(-1) |
0.47 |
3.59* |
GFCEG(-1) |
0.05 |
4.87* |
Adj R-squared |
0.36 |
|
DW |
1.64 |
|
SE of regression |
1.83 |
|
No. of Observations |
41 |
|
Note: t-statistics are based on HAC standard errors corrected with Newey-West/Bartlett window and 3 lags.
*: Significant at 5% level. |
Table 17a: Estimated impact of real WALR on Private Consumption Growth (PFCEG) |
|
Coefficient |
t-Statistic |
C |
2.88 |
2.66* |
WALR1QLAG(-1) |
-0.01 |
-0.07 |
PFCEG(-1) |
0.52 |
4.25* |
GFCEG(-1) |
0.05 |
4.95* |
R2 |
0.35 |
|
DW |
1.73 |
|
SE of regression |
1.86 |
|
No. of Observations |
41 |
|
Note: t-statistics are based on HAC standard errors corrected
with Newey-West/Bartlett window and 3 lags.
*: Significant at 5% level. |
Table 17b: Estimated impact of real WALR on Private Consumption Growth (PFCEG) |
|
Coefficient |
t-Statistic |
C |
2.99 |
1.82** |
WALR2YMA(-1) |
-0.02 |
-0.11 |
PFCEG(-1) |
0.52 |
4.23* |
GFCEG(-1) |
0.05 |
5.18* |
R2 |
0.35 |
|
DW |
1.72 |
|
SE of regression |
1.86 |
|
No. of Observations |
41 |
|
Note: t-statistics are based on HAC standard errors corrected with Newey-West/Bartlett window and 3 lags.
*, **: Significant at 5% and 10% level, respectively. |
Government expenditure, one would presume, should be sensitive to changes in interest rates, since interest payments account for a large share of government’s total and revenue expenditure (Chart-13). Because of higher interest rates, government expenditure should increase, unless the government also adopts contractionary fiscal policy to enhance the effectiveness of tight monetary policy, in which case lower government expenditure and higher interest rate may co-exist. Instead of overall government expenditure, this paper aims at assessing the sensitivity of government final consumption expenditure (GFCE), which is part of the demand side GDP. GFCE, however, excludes all transfers like interest and subsidy payments, and includes wages and salaries as a major component, which by nature are insensitive to interest rate changes. Estimated impact analysis also validates the perception. Even though the estimated coefficient of lending rate is negative, it is not statistically significant (Table 18).

Table 18: OLS estimates of impact of WALR on Government Consumption Growth (GFCE) |
|
Coefficient |
t-Statistic |
C |
25.99 |
1.54 |
WALR(-1) |
-2.07 |
-1.65 |
GROWTH |
0.69 |
1.42 |
D2008Q4 |
46.80 |
37.26* |
R2 |
0.31 |
|
DW |
2.41 |
|
SE of regression |
10.40 |
|
No. of Observations |
40 |
|
Note: t-statistics are based on HAC standard errors corrected with Newey-West/Bartlett window and 3 lags.
*: Significant at 5% level. |
Since higher interest rates cause lower GDP and non-agricultural GDP growth as per the estimates presented above, and given the fact that monetary policy aims at operating through aggregate demand (even though with lags it may also impact aggregate supply), how three major domestic components of demand respond to changes in interest rate remain a crucial empirical research issue. Because that OLS estimates do not capture the unknown dynamic interactions between the three components of demand, inflation and nominal lending rate, a VAR was used to study the impulse response path of all three demand components and inflation in response to the interest rate shock. Instead of real lending rate, nominal rate and inflation are taken as two variables in the VAR, given the two way causation between these two variable, i.e. higher inflation may drive nominal rates to rise, and higher rates may in turn lead to lower inflation.
 A mere look at the trends in nominal weighted average lending rates and four quarter moving average y-o-y growth in three components of domestic demand do not suggest any easily inferable causal relationship (Chart-14). In the VAR, however, all three components of demand seem to be sensitive to changes in interest rates, with the GFCEG being the most sensitive and PFCEG the least sensitive (Table 19 and Chart-15). Even if one assumes that GFCEG is more a reflection of the fiscal policy stance, for monetary policy to be effective, both private consumption and investment demand should be sensitive to changes in interest rate. It is possible that, as appears from previous estimates, PFCE may not exhibit direct sensitivity to changes in interest rate, but given the sensitivity of GFCF to interest rate, when investment declines in response to higher rates, that may lower income and employment prospects, which in turn may lower PFCE with a lag. Recent experience seems to suggest so, i.e. while growth in GFCF decelerated over successive quarters of 2011-12 and remained sluggish in the first half of 2012-13, PFCF showed stronger declaration only with lags, in the first half of 2012-13. The possibility of PFCF responding to changes in interest rate indirectly through changes in GFCF first is validated by estimates presented in Table 17.
Table 19: Quarterly and cumulative impact of 100 bps increase in WALR on demand |
|
D(LGFCE) |
D(LPFCE) |
D(LGFCF) |
DLWPI |
|
Quartely |
Cumulative |
Quartely |
Cumulative |
Quartely |
Cumulative |
Quartely |
Cumulative |
Q1 |
0.00 |
|
0.00 |
|
0.00 |
|
0.00 |
|
Q2 |
-1.60 |
-1.60 |
-0.14 |
-0.14 |
-0.17 |
-0.17 |
-0.34 |
-0.34 |
Q3 |
-0.55 |
-2.15 |
-0.08 |
-0.22 |
-0.23 |
-0.40 |
-0.49 |
-0.83 |
Q4 |
-1.04 |
-3.19 |
-0.01 |
-0.23 |
-0.35 |
-0.75 |
-0.54 |
-1.37 |
Q5 |
-0.71 |
-3.90 |
-0.02 |
-0.24 |
-0.32 |
-1.07 |
-0.53 |
-1.90 |
Q6 |
-0.76 |
-4.66 |
0.01 |
-0.24 |
-0.32 |
-1.39 |
-0.50 |
-2.41 |
Q7 |
-0.63 |
-5.29 |
0.00 |
-0.23 |
-0.29 |
-1.69 |
-0.46 |
-2.87 |
Q8 |
-0.60 |
-5.88 |
0.01 |
-0.22 |
-0.27 |
-1.96 |
-0.42 |
-3.29 |
Q9 |
-0.53 |
-6.41 |
0.01 |
-0.21 |
-0.25 |
-2.21 |
-0.38 |
-3.68 |
Q10 |
-0.48 |
-6.89 |
0.01 |
-0.21 |
-0.22 |
-2.43 |
-0.35 |
-4.02 |
Q11 |
-0.43 |
-7.31 |
0.01 |
-0.20 |
-0.20 |
-2.63 |
-0.31 |
-4.34 |
Q12 |
-0.38 |
-7.70 |
0.01 |
-0.19 |
-0.18 |
-2.81 |
-0.28 |
-4.62 |
Q13 |
-0.34 |
-8.04 |
0.01 |
-0.19 |
-0.16 |
-2.97 |
-0.25 |
-4.87 |
Q14 |
-0.31 |
-8.35 |
0.01 |
-0.18 |
-0.15 |
-3.12 |
-0.23 |
-5.09 |
Q15 |
-0.28 |
-8.63 |
0.01 |
-0.18 |
-0.13 |
-3.25 |
-0.20 |
-5.30 |
Q16 |
-0.25 |
-8.87 |
0.00 |
-0.17 |
-0.12 |
-3.37 |
-0.18 |
-5.48 |
Q17 |
-0.22 |
-9.10 |
0.00 |
-0.17 |
-0.11 |
-3.47 |
-0.16 |
-5.64 |
Q18 |
-0.20 |
-9.30 |
0.00 |
-0.16 |
-0.09 |
-3.57 |
-0.15 |
-5.79 |
Q19 |
-0.18 |
-9.48 |
0.00 |
-0.16 |
-0.08 |
-3.65 |
-0.13 |
-5.92 |
Q20 |
-0.16 |
-9.64 |
0.00 |
-0.16 |
-0.08 |
-3.73 |
-0.12 |
-6.04 |

Section-VI: Feedback from Consultations with CFOs of
Corporates and Commercial Banks
The assessment of the impact of changes in nominal and real interest rates on growth and investment is effectively an aggregation of the impact across different sectors, different projects, and different economic agents. In the absence of disaggregated detailed information, while one option could be to study firm level data across different sectors, the other option is to engage in discussions with senior representatives of corporates and banks who constantly analyze the interest rate scenario, often in a forward looking manner, for planning and executing investment projects. This section reflects the essence of the feedback that was received from the CFOs of about 15 corporates and commercial banks in a seminar held in the RBI in March 2013 on the role of interest rate in influencing economic activities.
The overall response suggested that nominal interest rate is more important than real interest rate for investment planning at the firm level, even though for the assessment of growth and investment at the macroeconomic level real interest rate could be relevant. At the firm level, both nominal cash flows from an investment project and nominal hurdle rate would invariably presume some underlying inflation expectations, but neither cash flows nor the hurdle rate may be explicitly evaluated in real terms. The second important feedback highlighted the role of expectations, since in an uncertain economic environment sharp changes in the outlook can alter expected cash flows significantly, which may be more important for an investment decision than the interest rate. The third major feedback was on the importance of leverage – i.e. debt equity ratio – which could vary significantly across sectors. In a highly leveraged sector, such as infrastructure, the required rate of return on equity may remain high, but the actual return on equity will be a function of interest costs and cash flows outlook. If interest costs rise and expected cash flows decline, arranging adequate equity capital could be difficult, which in turn may lead to shelving of some of the planned investment projects. In this context, many CFOs pointed out the significance of interest cost as percentage of sales and EBIDTA (earnings before deduction of interest, tax, depreciation and amortization expenses) than total costs. In a period of slowdown in growth and investment, interest costs as percentage of total costs may remain low, but because of the deceleration in growth of sales and lower EBIDTA, interest cost will be an important determinant of investment. Most corporates appeared to be facing cash flow problems due to: (a) sluggish demand conditions, (b) resultant weak pricing power, (c) still high input costs, and (d) significant delays in collection of receivables after delivery of orders.
Some of the CFOs underscored the need to look at indirect interest costs along with direct costs, since power and transportation costs may increase when interest rate rises. There was also a suggestion that the impact of high interest cost needs to be examined separately for small and medium firms, since the borrowing costs for start ups and SMEs may be higher than what is generally believed 6. The general feedback corroborated the standard central banking perception that inflation expectations matter for investment decisions, and by containing inflation and anchoring inflation expectations a central bank can contribute to stimulate investment and growth. There was a suggestion, however, to examine both demand and supply side effects while changing policy interest rates to manage inflation. While a lower interest rate may impact auto and housing sectors more directly, higher auto and housing demand can in turn improve demand for cement and steel, and in some of these sectors supply response could match the pickup in demand to avert any risks to inflation.
During the discussions it emerged that firms may have to be more careful while conducting sensitivity analysis before staring a project, since assuming the high growth or low inflation scenarios of a few years in the past to continue forever may not be appropriate. The risk premium implicit in the hurdle rate and projected cash flows from a new project should reflect realistic macroeconomic environment in which the project has to operate, which in turn can help stabilize the business cycle by dampening both extreme optimism and pessimism. The overall feedback from the consultations underscored the point that the debate on nominal versus real interest rate should be context specific, since both rates matter in an economy, with possibly greater relevance of nominal interest rate at the firm level for investment decisions and greater significance of the real interest rate at the aggregate macroeconomic level, where policy interest rate, market interest rates, and inflation expectations interact in a complex manner, but together influence real investment and growth.
Section-VII: Concluding Observations
The anti-inflationary monetary policy stance of the RBI, as reflected in the high nominal policy interest rate since mid-2011, has been generally perceived as one of the factors contributing to the sustained sluggishness in GDP growth and investment activity over past several successive quarters. For a period of more than eighteen successive months since July 26, 2011, the RBI’s repo rate was maintained at or above 8 per cent. While growth sacrifice as a means to contain inflationary pressures is context specific, depending on the drivers of inflation, it remains a debatable issue as to whether nominal or real interest rate matters for influencing growth and investment. Theoretical and empirical literature suggests that investment and growth could be sensitive to changes in both nominal and real interest rates. While economic decisions relating to investment, consumption and saving may be generally based on assessment of nominal interest rates and inflation, effectively, it is the real interest rate which matters. Consultations with representatives of industry and commercial banks revealed that nominal interest rate is more relevant for firm level investment decisions, but inflation expectations and the inflation environment also matter, pointing thereby to the implicit role of real interest rate in influencing investment. A central bank’s assessment of effectiveness of monetary policy generally takes into consideration the transmission of nominal policy rate changes through nominal market interest rates to ultimate goal variables, namely growth and inflation. However, given the argument that it is the real interest rate which matters for real variables like investment and growth, there is a view that nominal interest rate may not always reflect the correct stance of monetary policy. For example, if nominal policy rate is high, but both inflation and inflation expectations are also high, then despite a higher nominal policy rate, the implied real rate may suggest an expansionary, rather than an anti-inflationary stance of monetary policy.
If the level of the real interest rate is a better reflection of monetary policy stance, then how can a central bank influence the real interest rate? The review of literature presented in this paper suggests that a central bank can influence real rates through financial repression/reforms and lagged monetary policy response to inflation. Thus, real interest rate is a real phenomenon, but it could change in the short-run depending on how monetary policy responds to inflation and inflation expectations. In India, lack of one-for-one policy interest rate response to inflation - an evidence of lack of Fisher effect – explains partly the non-constant real rates over time. As highlighted in the paper, real lending rates in India in recent years have generally been lower than the rates that prevailed during the high growth phase before the global crisis. Thus, despite anti-inflationary monetary policy stance of nominal policy rate, lower real lending rates seemed to suggest the contrary. The key question that this paper then addresses is despite a lower real interest rate environment, why investment and growth weakened, instead of showing some acceleration? On this question, the paper is emphatic that when real interest rate declines, that may coincide with a phase of falling marginal productivity of capital at the aggregate level, and falling IRR at the firm level. In India, the incremental capital output ratio (ICOR) has increased in recent quarters, and correspondingly the implicit marginal productivity of investment has also declined. As a result, lower levels of real interest rate would have also contributed to the slowdown in growth. The next question that this paper explores is by how much the deceleration in growth and investment could result from a hypothetical 100 bps increase in the real interest rate? This is an empirical, country specific issue, and this paper employs alternative econometric methodologies to estimate the impact for India.
Both VAR impulse response and regression estimates suggest that for 100 bps increase in real interest rates, the decline in GDP/non-agricultural GDP growth could be about 20 to 23 bps. Panel regressions (for 24 sectors) and OLS regression estimates suggest that for 100 bps increase in real rates, investment rate may decline by about 50 bps. This result holds for both “investment to GDP ratio” and “growth in gross fixed capital formation”. Sensitivity of different sectors to changes in real rates do vary. Quantile regression results point to rising sensitivity of investment to nominal interest rate over higher quantiles, with the median impact for 100 bps increase in nominal effective borrowing costs coming to 88 basis points. In panel regression estimates, the impact of nominal rate on investment ranges between 62 bps to 91 bps. The estimated higher sensitivity of investment to nominal interest rate needs to be interpreted with caution, since nominal interest rate relates to nominal investment and real rate relates to real investment in the estimates. Among the three major components of domestic demand, government final consumption expenditure does not exhibit statistically significant sensitivity to changes in nominal lending rates. Private consumption expenditure also does not exhibit much sensitivity, reflecting weak dependence of consumption demand on credit and leverage. Thus, the monetary policy impact assessed through changes in lending rates suggest that the impact primarily operates through investment demand, as reflected in the growth of gross fixed capital formation (GFCF). Consumption demand, instead of being sensitive directly to interest rate, is sensitive to investment demand. When investment decelerates, that adversely impacts income and employment prospects, which in turn lowers consumption demand, with lags. This explains the longer transmission lags, and sharper deceleration in investment demand before consumption demand starts moderating in response to tight monetary policy. Since higher inflation tolerance is a means to lower real interest rate, this paper compares the favourable impact of lower real lending rate on growth with the adverse impact of inflation exceeding a threshold level of 6 percent on growth. Estimates suggest that the negative impact on non-agricultural GDP growth from higher inflation tolerance exceeds the positive impact of inflation induced lower real interest rate on growth. Thus, tolerating higher inflation with growth-supportive monetary policy response is unlikely to stimulate growth to the desired extent, since the adverse impact of higher inflation on growth would more than offset the favourable impact of growth-supportive monetary policy on growth. Adverse growth impact of high inflation is seen to operate primarily through compression of consumption demand, since investment demand, as per the estimates of this paper, is more sensitive to lower real rates than higher inflation. The major policy relevant finding of this paper is that lower real rates can stimulate growth, but lower real rates attained through higher inflation tolerance will lead to lower growth with higher inflation, and this unpleasant mix can be best avoided with an overall anti-inflationary thrust in monetary policy, which may accommodate needs of growth only when inflation remains contained below the threshold level of 6 per cent.
Overall, while this paper finds empirical evidence that lower real interest rates can stimulate growth and investment, it does not recommend a policy of higher inflation tolerance as the means to lower real rates.
Appendix
Appendix Table 1: Results of Unit root tests |
Variable |
Tests |
t-Stat/
LM Stat |
P-value |
Variable |
Tests |
t-Stat/
LM Stat |
P-value |
DLNAGDP |
ADF |
-8.4* |
0.0000 |
NFCGDP_SA |
ADF |
-0.8 |
0.9554 |
DLWPIC |
ADF |
-4.6* |
0.0004 |
D(NFCGDP_SA) |
ADF |
-6.0* |
0.0000 |
DLWGDP |
ADF |
-3.8* |
0.0052 |
GROWTH |
ADF |
-3.4* |
0.0145 |
DLGPF |
ADF |
-8.9* |
0.0000 |
GFCGDP_SA |
ADF |
-1.2 |
0.6883 |
WALR1 |
ADF |
-5.0* |
0.0015 |
D(GFCGDP_SA) |
ADF |
-9.8* |
0.0000 |
WALR1QLAG |
ADF |
-6.4* |
0.0000 |
GFCFG |
PP |
-4.0* |
0.0024 |
WALR2YMA |
ADF |
-3.4** |
0.0601 |
GFCE |
ADF |
-7.4* |
0.0000 |
WALR2YMA |
KPSS |
0.08 |
|
PFCE |
ADF |
-3.0* |
0.0383 |
GDPG |
ADF |
-3.2* |
0.0225 |
D(LGFCE) |
ADF |
-10.3* |
0.0000 |
GFD |
ADF |
-2.8** |
0.0642 |
D(LGFCF) |
ADF |
-9.6* |
0.0000 |
GFD |
PP |
-6.5* |
0.0000 |
D(LPFCE) |
ADF |
-11.3* |
0.0000 |
WGDP |
ADF |
-5.3* |
0.0001 |
D(LWPI) |
ADF |
-5.4* |
0.0000 |
INFLTR |
ADF |
-5.7* |
0.0000 |
GSEC10Y |
KPSS |
0.5 |
|
NAG |
ADF |
-2.9* |
0.0540 |
|
|
|
|
Note: *, **: significant at 5% and 10%, levels, respectively.
Null hypothesis in ADF and PP test: Series is non-stationary and in KPSS test: Series is stationary |
Data and Variables
For the panel analysis, data for 24 sectors, stratified from a sample of common set of companies over the period 2000-01 to 2010-11 were collected for the variables gross fixed assets (GFA), interest payment, borrowings, sales, profits before interest and tax (PBIT) from company-wise balance sheet data available with the Reserve Bank. Growth in gross fixed assets (GFAG) is used as a proxy of investment, effective cost of debt capital (COST), derived from interest payments and borrowings, is used as a proxy of nominal lending rate, PBIT to sales ratio (PBITSAL) and sales growth (SALG) as a proxy of economic activity are the key variables in the for panel regressions. For macro level analysis, data are collected from Central Statistics Office, Reserve Bank’s Database on Indian Economy, Basic Statistical Returns and IMF online Database on GDP and its various components (both nominal and real), world GDP growth, WPI, non-food credit (NFC) and weighted average lending rate (WALR). Real lending rate is constructed taking the difference between WALR and backward looking measures of inflation expectations. Either one quarter lagged y-o-y WPI inflation or 2 year moving average of monthly y-o-y WPI inflation is used as a proxy for inflation expectations.
References:
Allsop, C. and Glyn A. (1999). “The assessment: real interest rates”, Oxford Review of Economic Policy, 15, 2, 1-16.
Chadha, Jagjit S. and Dimsdale, Nicholas H. (1999). “A long view of real rates”, Oxford Review of Economic Policy, 15, 2, 17-45.
Deputies of the Group of Ten (1995), ‘Saving, Investment, and Real Interest Rates’, A study for the Ministers and Governors, October.
Fama, E.F. (1975). “Short-term interest rates as predictors of inflation”, American Economic Review, 65(3), 269-282.
Fisher, Irving (1936). The theory of interest, New York, Augustus M. Kelley.
Friedman, M. and Schwartz, A.J. (1976). “From Gibson to Fisher”, Exploration in Economic Research, 3, 288-291.
Fry, M.J. (1997). “In favour of financial liberalization”, Economic Journal, 107, 442, 1-16.
Geng, Nan and N’Diaye Papa (2012). “Determinants of corporate investment in China: Evidence from cross-country firm level data”, IMF Working Paper No. WP/ 12/80, International Monetary Fund.
Hafer, R.W. and Hein, Scott E. (1982). “Monetary policy and short-term real rates of interest”, Federal Reserve Bank of St. Louis, 13-19.
Huizinga, John and Mishkin, Frederic S. (1986). “Monetary policy regime shifts and the unusual behavior of real interest rates”, Camegie-Rochester Conference Series on Public Policy,24, 231-274.
IMF (2013), “India 2013 Article IV Consultation”, IMF Country Report No. 13/37, February 2013.
Keynes, J.M. (1936). The General Theory of Employment. Interest and Money, Macmillian, London.
Mallick, Hrushikesh and Agarwal, Shashi (2007). “Impact of real interest rates on real output growth in India: A long-run analysis in a liberalized financial regime”, The Singapore Economic Review, 52, 2, 215-231.
Manopimoke, Pym (2008). “An analysis of the real interest rate and monetary policy under regime shift”, url: http://www.apeaweb.org/confer/bei08/papers/mano_p.pdf.
Marshall, Alfred (1890). Principles of Economics, Macmillian, London.
Mckinnon, R. (1973). Money and Capital in Economic Development, Washington, D.C., Brookings Institute.
Mehrara, Mohsen and Karsalari, Abbas Rezazadeh (2011). “The nonlinear relationship between private investment and real interest rates based on Dynamic Threshold panel: The case of developing countries”, Journal of Money, Investment and Banking, 21, 32-42.
Mishkin, Frederic S. (1981). “The real interest rate: An empirical investigation”, Camegie-Rochester Conference Series on Public Policy, 15, 151-200.
Mishkin, Frederic S. (1984). “The real interest rate: a multi-country empirical study”, Canadian Journal of Economics, 17,2, 283-311.
Mohanty, D., Chakraborty A.B. and Gangadaran S. (2012). “Measures of nominal and real effective lending rates of banks in India”, RBI Working Paper (DEPR): 7/2012, May.
Mohanty, D., Chakraborty, A .B., Das, A. and John, J. (2011). “Inflation Threshold in India: An Empirical Investigation”, RBI Working Paper ( DEPR): 18/2011, September.
Moore, B.J. (1988). “Horizontalists and verticalists: The macroeconomics of credit money”, Cambridge, Cambridge University Press.
Pattanaik, Sitikantha and Nadhanael, G.V. (2011). “Why persistent high inflation impedes growth? An empirical assessment of threshold level of inflation for India”, RBI Working Paper ( DEPR): 17/2011, September.
Pill, H. (1997). “Real interest rates and growth: Improvement on some deflating experiences”, The Journal of Development Studies, 34, 1, 85-110.
Pill, H. and Pradhan, M. (1995). “Financial indicators and financial changes in Africa and Asia”, IMF Working Paper No. WP/95/123, International Monetary Fund.
Reinhart, Carmen (2012). “The return of financial repression”, CEPR Discussion Paper No. DP8947.
Shaw, E. (1973). Financial Deepening in Economic Development, New York, Oxford University Press.
Smithin, John N. (2003). Controversies in Monetary Economics,Edward Elgar Publishing Limited, Cheltenham, UK.
Stiglitz, Joseph E. (1999). “Interest rates, risks and imperfect markets: Puzzles and policies”, Oxford Review of Economic Policy, 15, 2, 59-76.
Summers, L.H. (1982). “The non-adjustment of nominal interest rates: a study of the Fisher effect”, NEBR Working Paper No. 836, National Bureau of Economic Research.
Taylor, P. Mark (1999). “Real interest rates and macroeconomic activity”, Oxford Review of Economic Policy, 15, 2, 95-113.
Thornton, Henry (1802). An inquiry into the nature and effects of the paper credit of Great Britain, Augustus M. Kelley, New York.
Tokuoka, Kiichi (2012), “ Does the Business Environment Affect Corporate Investment in India?”, IMF Working Paper, WP/12/70.
Tymoigne, Eric (2006). “Fisher’s theory of interest rates and the notion of “Real”: A critique”, Working Paper No. 483, The Levy Economics Institute, 1-34.
Upper, Christian and Worms Andreas (2009). “Real long-term interest rates and monetary policy: A cross-country perspective”, BIS Papers No. 19, Bank of International Settlement.
Wicksell, Knut (1907). “The influence of the rate of interest on prices”, Economic Journal, 17, 213-220.
Wray, L.R. (1992). “Alternative approaches to money and interest rate”, Journal of Economic Issues, 26, 1-33.
|